#ohms law

Tech Tips:

This tech tip recaps the livestream by the same name, featuring HVAC School contributors Matt Bruner and Adam Mufich, as well as special guests Craig Migliaccio (AC Service Tech) and Ty Branaman (love2hvac). You can watch that live stream on our YouTube channel HERE. Craig and Ty are HVAC educators with YouTube channels of their […]

Mistakes in Measuring Ohms

In HVAC and electrical school, one of the first things you learn about electricity is Ohm's law: Volts (V) = Amps (I) x Ohms (R) Pretty simple, right? Watt's law is just as easy: Watts (P) = Volts (E) x Amps (I) With this newfound knowledge, the student walks confidently into the real world with […]

What is Inductive Reactance?

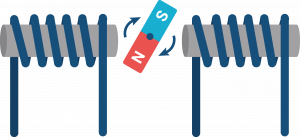
Ohm’s law is pretty straightforward; you multiply ohms by amps to get the voltage. Using variable E to represent voltage, variable I for amps, and variable R for ohms, the equation for Ohm’s law looks like this: E = I × R You can figure out the number of amps in a system using basic […]

Check out our most recent video

How to Evacuate an AC system, Full Vacuum Procedure

Our latest Podcast

HVAC School – For Techs, By Techs

Real training for HVAC ( Heating, Ventilation, Air Conditioning and Refrigeration) Technicians. Including recorded tech training, interviews, diagnostics and general conversations about the trade.

Chris Hughes and Steve Rogers from The Energy Conservatory discuss TEC’s TrueFlow technology and its crucial role in predicting airflow issues in the sales process, particularly for heat pump retrofits. They explain how the TrueFlow app helps contractors accurately measure both static pressure and airflow in existing systems, enabling them to predict whether proposed equipment changes will work effectively. This is especially vital when considering the growing trend of replacing gas furnaces with heat pumps, as the airflow requirements can differ significantly.

The conversation delves into a detailed example of replacing an 80% efficient 48,000 BTU furnace with a heat pump. They demonstrate how the airflow requirement would nearly double from 652 CFM to 1,200 CFM, potentially causing the static pressure to quadruple due to Fan Law 2 principles. This dramatic increase could lead to significant performance issues if not properly addressed during the system design phase. The experts emphasize that using TrueFlow during the sales process allows contractors to identify potential problems and necessary modifications before installation rather than discovering issues during callbacks.

The discussion also covers the ideal workflow for system assessment and sales, starting with customer comfort evaluation and proper load calculations. It’s important to reduce loads through envelope improvements when possible, particularly in cold climates where heat pump retrofits are becoming more common. Proper system sizing often means going smaller rather than larger, contrary to common industry practices, and the worst HVAC systems are often found in expensive custom homes rather than tract housing.

Key Topics Covered:

TrueFlow technology and its role in system forecasting
Fan Law 2 and its impact on static pressure when changing airflow
Heat pump retrofit challenges and considerations
The importance of accurate load calculations
Dual fuel systems as a solution for cold climates
The relationship between airflow and static pressure
Sales process integration of technical measurements
Common issues with oversized equipment
The importance of envelope assessment
Methods for reducing system loads
Proper duct sizing and modification strategies
The role of proper filter sizing and selection
The benefits of flat-rate pricing for duct modifications
Training resources and support available for TrueFlow users

Check out TEC’s YouTube channel at https://www.youtube.com/@energyconservatory, and you can visit TEC’s website at https://energyconservatory.com/.

Have a question that you want us to answer on the podcast? Submit your questions at https://www.speakpipe.com/hvacschool.

Purchase your tickets or learn more about the 6th Annual HVACR Training Symposium at https://hvacrschool.com/symposium. Subscribe to our podcast on your iPhone or Android. Subscribe to our YouTube channel. Check out our handy calculators here or on the HVAC School Mobile App for Apple and Android.